Parallel Circuits

  Parallel Circuits 

When current flows along more than one path, how does it get divided up?
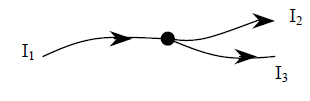
So far, we have considered circuits in which the current flowed along only a single path. The charges never “had a choice” about where to go. However, most circuits are a lot more complicated than that. There are multiple paths along which charge may flow, and the geometrical layout of the circuit can get very complex. A location at which current may branch off into two or more different paths is called a junction. There is a simple and important rule that governs the behavior of current at such a junction. It is called Kirchoff’s Junction Rule: The total current flowing into a junction equals the total current flowing out of that same junction. The explanation for this rule is also simple: if the current flowing out were less than the current flowing in, that would mean that charges were getting trapped in the junction. Eventually (and actually, very very quickly) the pileup of charge would cause a meltdown in the junction. If, on the other hand, the current flowing out were more than the current flowing in, that would mean that there was some external source of charge putting extra charges into the junction. We assume that that never happens. So for instance, for the diagram shown here, we have the relationship

$I_{1}=I_{2}+I_{3}$

Now we can examine the simplest circuit in which current can flow along more than one path: a two-resistor ``parallel`` circuit. (It’s called a parallel circuit because of the geometry of the circuit diagram.)

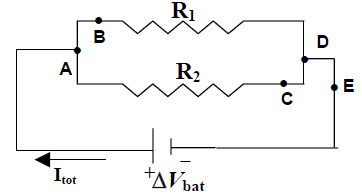
In this circuit, current flows out of the battery from the positive terminal, and then divides at point A. Part of the current goes through $R_{1}$
, and part flows through $R_{2}$
. Then, at point D, the current combines again. Let’s assume that $R_{2}=2R_{1}$ , and answer these questions.
(1) What is the ranking of the potential at the points A, B, C, D and E?
Answer: $V_{A}=V_{B}>V_{C}=V_{D}=V_{E}$ . Points A and B are connected by “perfectly conducting” wires to the positive terminal of the battery, so the potential at those points must be the same as that at the positive terminal. Similarly, points C, D, and E are connected to the negative terminal of the battery, and so must all be at the same potential. (We will always assume that the negative terminal of the battery is at a potential of zero volts.)
Question: What is the relationship between I1 (the current flowing through resistor $R_{1}$) and $I_{2}$ (the current flowing through resistor $R_{2}$)?
Answer: First we need to realize that $\Delta V_{1}=\Delta V_{2}=\Delta V_{battery}$ . This is because all three of those potential differences are equal to $\Delta V_{AD}=\Delta V_{A}-\Delta V_{D}$ , which we could ParallelCircuitsFigure out from the ranking of potentials given above. Now, we know that I1 = V1  R1. Also, we can see that $I_{2}={\frac {\Delta V_{2}}{R_{2}}}={\frac {\Delta V_{1}}{R_{2}}}={\frac {\Delta V_{1}}{2R_{1}}}={\frac {1}{2}}\Delta V_{1}={\frac {1}{2}}I_{1}$ . So our final result is that $I_{2}={\frac {1}{2}}I_{1}$ . More current flows through the smaller resistor.

What is the ranking of the current flowing past points B, C and E?
Answer: $I_{E}>I_{B}>I_{C}$ .
All of the current – that is, $I_{total}$ – must flow through point E. The amount flowing past point B is the same as the amount flowing through resistor $R_{1}$

(i.e., $I_{1}$ 

) while that flowing past point C is the same as $I_{2}$
.
Finally, we need to give a definition of “equivalent resistance,” symbolized by $R_{equiv}$. The equivalent resistance is like an imaginary “substitute” resistor that replaces all of the resistors in a particular circuit. We can imagine putting all of the resistors in a circuit – with all of their complicated connections, which may combine both series and parallel connections – in a closed “black box,” with one wire going in, and one wire going out. When we hook up that black box to a battery, we get a certain amount of current flowing out of the battery, which we call $I_{total}$ where $I_{total}={\frac {\Delta V_{battery}}{R_{BlackBox}}}$ . Then we see that $R_{BlackBox}={\frac {\Delta V_{battery}}{I_{total}}}$ . Suppose we want to take away that black box with all of its complicated connections, and replace it with one single resistor – but we want to use the same battery, and get the same $I_{total}$ as before. Then that single resistor should have a resistance equal to $R_{equiv}$, where we choose $R_{equiv}$ so that we get the same current as we did with the black box. Therefore, for any circuit, $R_{equiv}={\frac {\Delta V_{battery}}{I_{total}}}$ .