Module : Applications of Newton' Laws : Friction

Kreshnik Angoni, and Kevin Lenton
Word Worksheet
PDF Worksheet

Other Resources:

• Haliday & Resnick, Fundamentals of Physics 6.1-4
• Openstax

Learning Objectives

After reading this page, watching the videos and reviewing the exercises, you will be able to:

• Understand what causes friction forces.
• Know the formulas for static and kinetic friction.
• Know to avoid some common misconceptions.
• Apply the concepts to some dynamics problems.

Basic Definition

Friction is a resistive force, which resists the sliding (kinetic friction) or attempted sliding (static friction) of two surfaces.

Tribology

Tribology is the science of 'interacting surfaces in relative motion'. It includes the study of friction, wear, lubrication, etc.
Any surface that appears smooth, is really quite rough when looked at very closely. See this image below from the McGill Materials Engineering Group:
https://www.mcgill.ca/materials/files/materials/sem-images.png
Imagine two of these surfaces pushed together, and then made to slide over top of each other.
Friction is caused by forces between points where surfaces meet. If two glass plates are made really flat and polished and cleaned and made to touch in a vacuum, they bond together. It would look as if the glass was just one piece. The bonds are formed as in a normal glass piece. This is called cold welding. And this is the main cause of friction.
The detailed microscopics of friction are complicated. However, by focusing on practical details we can arrive at useful Models of Friction.

Some observations:

• Heavier objects have more friction than lighter ones
• On surfaces with friction it usually takes more force to get an object moving than keep it moving
• It is harder to move objects on rough surfaces than smooth ones

Friction should be proportional to the force the surface exerts on another surface, which is of course the normal force ${\vec {N}}$ . As an equation this means that the magnitude of the frictional force can be expressed as

$|{\vec {F}}_{friction}|=\mu |{\vec {N}}|$

and we can also suppose we will need different constants for a stationary object compared to a moving one. i.e we have different coefficients of static friction (μs) and kinetic friction (μk).

Remember that the normal force is perpendicular to the surface. Frictional forces are always parallel to the surface.

The Coefficient of Friction $\mu$

• The coefficient is always positive. A negative coefficient would imply the force of friction is assistive (adds energy) rather than resistive (removes energy from the system).
  
• The coefficient has no units. It is just a number.
• Coefficients of friction usually have values between 0.3 and 0.6. However, teflon can have a coefficient as low as 0.04. Rubber-coated surfaces can have a coefficient of friction substantially larger than 1.
  

How much force does it take to separate two interleaved phone books?

Second part of video: Worth Watching

Pushing a book on the Table

Perform a short experiment by pushing a book on the table with an ever increasing horizontal force. The book does not move initially, even though you are pushing the book. The force of static friction is matching the force with which you are pushing on it. Eventually you overcome the force of static friction and the book starts to slide. The sliding is opposed by the force of kinetic friction, which then remains roughly constant.
The maximum static friction is always greater than the kinetic friction. This means the coefficient of static friction is always greater than the coefficient of kinetic friction.
This is shown in this figure showing the frictional forces as a function of the applied force:

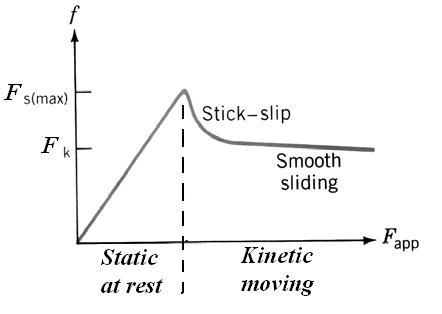
This is explained in this video.
Static and kinetic Friction

Force of Static Friction

For static friction, the force of friction matches the force applied, keeping the surfaces from sliding. When the applied force exceeds the maximum static frictional force, the surfaces will begin to slide. The maximum frictional force is calculated as follows:

$|{\vec {F}}_{static}|\leq \mu _{static}|{\vec {N}}|$

The static frictional force is less than or equal to the coefficient of static friction times the normal force. Once the frictional force exceeds this value, the surfaces will break away and begin to slide.

Force of Kinetic Friction

Once it is moving, the frictional force then obeys:

$|{\vec {F}}_{kinetic}|=\mu _{kinetic}|{\vec {N}}|$

Note that once moving the force of kinetic friction is constant; that is to say it has the same value whatever velocity the object is moving at.

Misconception #1: Fs= mu N

Students can sometimes be heard saying:
"The force of static friction is equal to $\mu _{s}|{\vec {N}}|$ "
Be very careful with this because the formula gives the limit or max for the force of static friction.
$|{\vec {F}}_{static}|\leq \mu _{static}|{\vec {N}}|$
"The force of static friction is less than or equal to $\mu _{s}|{\vec {N}}|$"
If the applied horizontal force on the above book is less than this limit, the force of static friction will be equal in magnitude, but opposite in direction, to the applied force.
For instance, let's say the maximum the static friction can be is 30N (Ok, ok it's a big book).
You apply 10N horizontally, the static friction matches it to 10N.
You apply 20N, the static friction matches it to 20N.
You apply 30N, the static friction matches it to 30N.
You apply 31N, the maximum static friction is exceeded and the surface starts to slide. The force of kinetic friction is now in operation.
This misconception is not helped by the fact that many textbook problems ask questions at this limit, just so you can use the equation.
This is explained in this video.
Static Friction

Misconception #2: Friction always opposes motion

You will often see "friction always opposes the motion" on websites and even in textbooks. This is not true, if you just say it like that. What is true is that friction always opposes the sliding or the attempted sliding.
The classic case for this is the tires of a car on the road, moving forward. First off, assume the wheels are not spinning: the friction is static friction, the rubber is not sliding on the road.
If the road were covered in ice (no friction), how would the wheels spin? They would push back on the road.
This means the road pushes forward on the wheels of the car (Newton 3). This is because friction always involves two surfaces and you have to 'frictionize' both surfaces.
So what direction is the force of static friction on the car? It pushes the car forward. What direction is the car moving? Also forward.

You can use similar arguments to show that the force of static friction pushes you forward when you walk forward.

Case of Friction on an Inclined Plane

Consider a block simply sitting on an inclined plane. If the plane were covered in ice, the block would slide down. Therefore, it is the force of friction, static friction, which is keeping it there.
The acceleration is zero, so the net force on the block must also be zero.
We can use a free body diagram and Newton's second law to determine the value of the force.

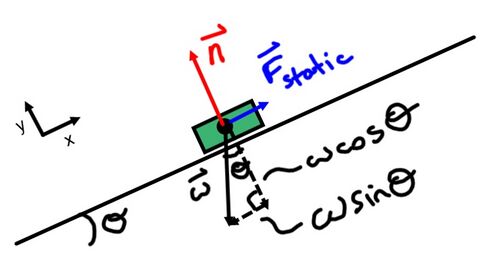
X Axis	Y Axis
$\sum F_{x}=ma_{x}$ $F_{staticfriction}-mgsin\theta =ma_{x}$ But the acceleration is zero $F_{staticfriction}-mgsin\theta =0$ $F_{staticfriction}=mgsin\theta$	$\sum F_{y}=ma_{y}$ $n-mgcos\theta =ma_{y}$ But the acceleration is zero $n-mgcos\theta =0$ $n=mgcos\theta$

Therefore the required force of static friction is $F_{staticfriction}=mgsin\theta$
and the normal force is
$n=mgcos\theta$
You can see that these are dependent on the angle. The bigger the angle, the bigger the required force of static friction, and the smaller the normal force. As shown in this diagram:

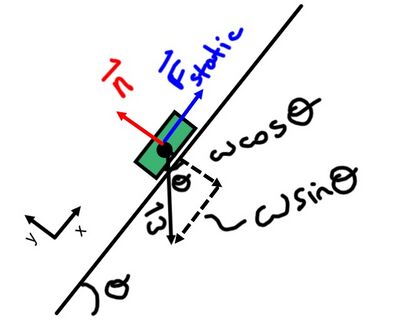
What happens if the angle gets too big?
If the x component of the weight exceeds the maximum possible force of static friction, the block will start to slide, and the force of kinetic friction will be in play.
If the block wants to slide up (e.g. because of an applied force or a previously applied force has made it move that way), the force of friction will be down the slope, if the block wants to slide down, the force of friction will be up the slope.
Remember, the force of friction always opposes the sliding motion, or the attempted sliding motion.

Examples

Example 1:
A block is sliding down an incline with angle $\theta$. The mass of the block is m. The coefficient of kinetic friction is equal to $\mu _{k}$. Find the acceleration of the block.
(Ans:$a=gsin\theta -\mu _{k}gcos\theta$)
Bonus: At what angle will the block slide with constant velocity?
Video Solution